There’s a well-known math-related meme that’s been going around the internet for years. It’s an image of a newspaper clipping with the headline: “‘We hate math,’ say 4 in 10—a majority of Americans.” In the meme, the word “majority” is highlighted, just in case readers don’t get the blunder.
There’s some debate about whether the clipping is real or not, but if we cast aside doubt and consider the article legit, it does reveal a couple of truths: Number one, there are a lot of people who really don’t care for mathematics (polls reveal the 40 percent number is about right); and, number two, there are a lot of people—newspaper copy editors included—who are really bad at it.
Even mathematicians will tell you that mathematics has a bit of an image problem, particularly in the U.S.
To the unenlightened, mathematics is an enigma, a mysterious world of theorems and proofs and peculiar-looking squiggly characters that don’t appear to have anything to do with numbers at all.
Mathematics represents the last letter in STEM—science, technology, engineering, and math—so it obviously has something to do with science and technology, right? Oftentimes, yes. But pure mathematics, the kind that’s done for its own sake, well, perhaps not.
And, actually, it’s not just math that many people don’t quite comprehend. It’s mathematicians themselves. Ask someone what a mathematician does and they’ll likely conjure up Hollywood-esque images of men—almost always men—with bad hair and rumpled clothing frantically scribbling unintelligible formulas on a chalkboard.
“Tell someone you’re a mathematician and they’re already too scared to ask a follow-up question,” admits Emily Riehl, an assistant professor in the Krieger School’s Department of Mathematics.
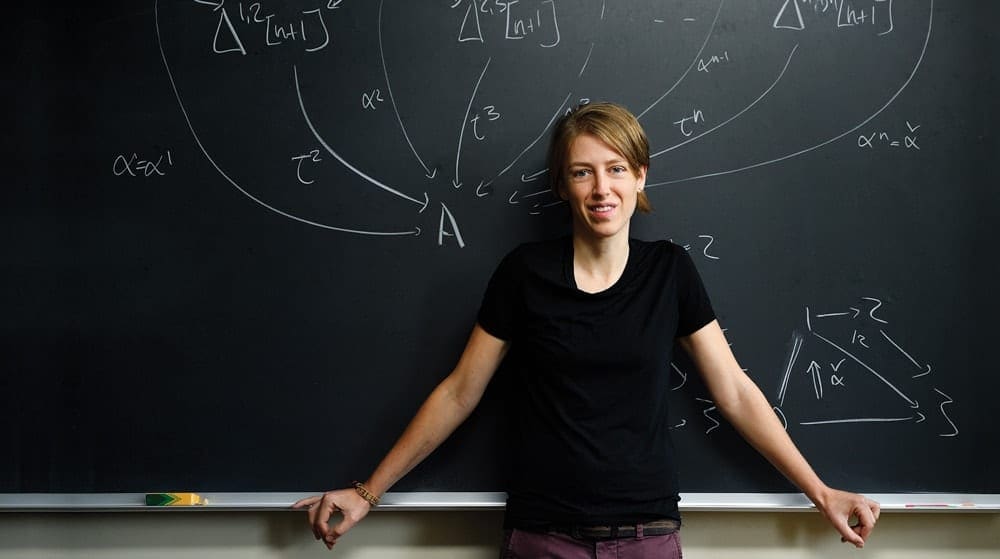
For assistant professor Emily Riehl, mathematics can be a very social endeavor. All of her recent papers have been collaboratively written.
But if you ask a mathematician to describe what mathematics is all about, their answer might surprise you. They’ll compare it to art. Or music. Or philosophy. Or any of the seemingly more “arts-oriented” pursuits in the arts and sciences canon. And despite how concrete and absolute mathematics appears on the surface, mathematicians say their field is about as abstract a subject as you can imagine.
“The idea of abstraction is so natural to a mathematician,” says Professor Nitu Kitchloo. “Math begins with intuition. We take something completely concrete, and then we make it so abstract it might be unrecognizable. We play with toys we can’t even picture. But this abstraction allows us to draw connections that may not have been evident before.”
Kitchloo, who served as chair of the mathematics department at the Krieger School from 2014 until last summer, doesn’t spend his days in front of a blackboard scribbling. Rather, when he isn’t teaching or meeting with students, you can find Kitchloo at a cafe. He admits he’ll spend hours there, nursing a coffee or tea, and staring off into space, deep in thought, just like a philosopher working on an argument or a poet thinking up his next line. What he’s doing is trying to get a feeling for the object he’s studying. In his case, Kitchloo studies topology, the intersection of geometric forms and spatial relations.
“In studying these objects, you often start with a glimpse of a small part of the bigger picture,” he says. “It’s like a tree and all you can see are the roots, but you’re trying to speculate on what’s up there: the leaves, the fruits … You have a limited amount of information, but you have a sense of what it might be like based on what you’ve seen in other trees of a similar nature.”
He needs no computer while he works—just a pencil and a piece of paper on which he’ll scribble a few calculations to see if reality agrees with his intuition. “One thing that sets pure mathematicians apart from others in STEM fields is that we don’t need to be tied to a physical location, encumbered by equipment and labs. We are much more similar to humanists in that way.”
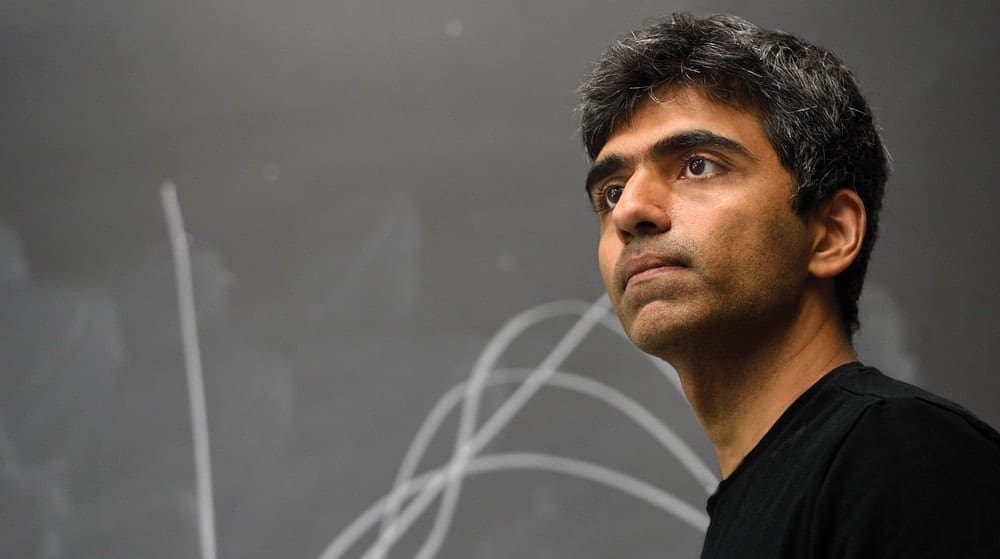
Professor Nitu Kitchloo says the idea of abstraction is natural to mathematicians.
Or as Kitchloo’s colleague Richard Brown puts it: “Mathematics is the music of reason.” He’s quoting the famed 19th-century English-born mathematician and professor, James Joseph Sylvester, who helped establish the mathematics department at Johns Hopkins in 1876. “Just like music, math is built on a very rigidly defined set of rules and our mathematical constructions exhibit an infinite amount of creativity.”
Brown, an associate teaching professor and director of undergraduate studies for the department, arrived at the Krieger School in 2005. At that time, there were approximately 50 undergraduate students majoring in math. Now the program boasts almost 80, along with another 1,500-plus undergraduates who take math classes annually. “We’re a strange department that way,” Brown says. “We’re relatively small, yet we have to service everybody in all their math courses.”
Brown originally thought he wanted to be an architect, someone who designs structures. But after also studying physics and working in industry for several years, he realized mathematical constructions, not physical ones, were his calling. As he told an audience at a 2014 TEDx Talk he dubbed “Why Mathematics?”: “To be really honest, math isn’t really about numbers. … It’s the ideas behind the numbers. It’s about logical structure.” Like architecture.
He says many freshmen who stop by his office don’t realize what advanced mathematics is all about because primary and secondary schools don’t expose them to the ideas behind the numbers. Formulas and algorithms are delivered with no context or motivation, with students made to simply memorize and apply them, at the expense of actual deep understanding of the naturally fascinating things mathematicians explore.
“There is a sense with mathematicians that we look at mathematics differently than the way high school teachers and students look at math,” says Brown, whose area of expertise is Hamiltonian dynamic systems, which deal with the more classical kind of mathematical models found in physics or engineering. “They tend to see math as only helpful for other subjects, as something that is utilitarian in use but not anything that anybody would consider beautiful or elegant or interesting in itself. In that sense, the way we teach pre-college math really shorts the student from seeing what math is really like.”
Recent graduate Thomas Brazelton ’18, former co-president of the Johns Hopkins Mathematics Club, Ex Numera, agrees. “I think the way that mathematics is taught in K-12, it pushes out a lot of people who would really love it if they were able to continue further,” he says. “Mathematicians tend to treat math as an art and really value creativity and creative insight. All of that is really stripped away the way it’s taught in K-12 education.”

Richard Brown (r), associate teaching professor, explains a mathematical concept to his class of undergraduates.
Brazelton, who is pursuing a doctoral degree in mathematics at the University of Pennsylvania, originally majored in international relations at Johns Hopkins. But then he took an elementary number theory class and was hooked. “It just had this intrinsic beauty that made it so rewarding to study,” he says. “With mathematics, you start with fundamental ideas you accept as axioms, and from there you can build anything you want. It’s very cool.”
“Math is pretty hard to talk about.”
Translating mathematical discoveries can also pose a challenge for mathematicians. Concepts are complicated and can’t be communicated in easy-to-digest soundbites. Math rarely makes the news—unless there’s some earth-shattering breakthrough, such as in 1995, when British mathematician Andrew Wiles developed the first successful proof for Pierre de Fermat’s so-called Last Theorem—something that had baffled mathematicians for 350 years. Or in 2014, when Maryam Mirzakhani was the first woman to receive the Fields Medal, the most prestigious honor in mathematics. Or in 2003, when Russian mathematician Grigori Perelman solved the Poincare Conjecture, which deals with shapes that exist in four or more dimensions. The general public likely still has no concept of what the conjecture involves, but it made the news because it’s one of the so-called Seven Millennium Prize Problems, which brings a million-dollar prize for each solution. (Perelman declined the money.)
Sometimes mathematicians even have trouble explaining their research to each other. Ask David Savitt, the new chair of the mathematics department, and he’ll tell you he works on algebraic number theory. And while other mathematicians know exactly what algebraic number theory is, when he tells them he’s specifically interested in Galois representations and p-adic Hodge theory, they might not be as sure. “In pure mathematics things get so specialized, that two or three steps away, people have only a vague idea of what you’re talking about,” Savitt says.
But despite the fact that theoretical mathematics is a highly specialized study, with academics doing most of the heavy lifting within their own minds, it can also be a highly collaborative endeavor.
“The field is much more social than I ever expected,” says Emily Riehl, who studies category theory, which is essentially the patterns of mathematical ideas and why they keep showing up in different contexts. She notes that all of her recent papers have been collaboratively written. “Math is pretty hard to talk about, so we’ll go halfway around the world to find somebody to have a conversation with.”
Kitchloo, the coffee-shop thinker, relishes the collaborative aspect. “Since every mathematician has their own unique flavor of intuition, when you meet another mathematician, chances are your intuitions don’t overlap that much, so you’ve just doubled your ability to solve that problem. You may be seeing half of it and they may see the other half. Put them together and—boom!—you’ve just solved the problem.”
In Search of Diversity
Another challenge for mathematicians is expanding diversity in their ranks. Like other STEM fields, mathematics has always had its challenges attracting women and minorities to the field, but the trend has been changing in recent years. In 2014, women’s representation in mathematics and statistics reached more than 40 percent at the bachelor’s and master’s levels nationally, but remained below 30 percent at the doctoral level. At the Krieger School, four of the math department’s 19 tenured or tenure-track faculty are women, which is a higher percentage than at many research universities. But there are no African-American faculty members.
Riehl says being female in a male-dominated field has had its pluses and minuses. It meant that people learned who she was at a younger age, but she believes that female math professors sometimes get less respect in the classroom. “There’s just something about the fact that people aren’t used to having a mathematical authority be female,” says Riehl.
She says she doesn’t think gender has been an issue in her dealings with colleagues at the university level, but she still hears from female students who have experienced bias in the past. “It’s surprising to me how many of my female students talk about people who have been explicitly discouraging or dismissive of them,” she says. “The default assumption is that they can’t be good at math, not that they can. But if we get out of the way of talented people who have been discouraged for years, that would do a lot to help the situation … It’s certainly changing. You can see at the research level there are a lot more women in my age cohort than a generation older.”
At the Krieger School, Mira Wattal and Emily Quinan, both junior math majors, started the Inclusivity in Mathematics Initiative last year to provide a place where underrepresented students would feel welcome. The group aims to survey every student at the university who has taken an undergraduate math class and learn about their experiences, as they relate to gender and ethnicity. They plan to release the results in a report they hope will spur greater awareness about the issue.
“Mathematics has traditionally been very much a boys’ club and can feel exclusionary,” says Wattal, who notes she has been the only female in several of her math classes. “There wasn’t a space for people who were underrepresented, so we wanted to create that space.”
Quinan calls the lack of diversity in mathematics a “pipeline issue,” something that starts in elementary school, when girls and minorities can experience an implicit bias about what groups should or shouldn’t be good at. Though Quinan says she’s had excellent female role models throughout her education, many people don’t. “It’s hard to sit in a class and not see anybody that looks like you,” she says. “Seeing into the future and thinking, ‘I’m not welcome in this field’ or ‘I’m not going to feel comfortable in this department’ might turn away people who are otherwise interested.”
A Valuable Commodity
Despite some challenges in the field, mathematics remains a compelling and popular area of study. Students know a mathematics major is a valuable commodity when it comes to looking for a job. At Hopkins, math majors can choose to concentrate in either applied mathematics via the Whiting School of Engineering—a popular double major for students interested in physics, engineering, or computer science—or “pure” math at the Krieger School.
“Human resources recruiters really value mathematics,” says Brown, who admits he was once hired by an engineering firm primarily because of his mathematics degree. “A math degree really teaches you how to think analytically and reason deductively, how to understand the logical structure of complicated things. In that guise, it’s really a great secondary degree for anything.”
Recent grads pursue fields as diverse as law, finance, and medicine. And some students, like Wattal, simply want to make studying math’s intrinsic beauty their life’s work.
Wattal says she entered the Krieger School as a physics major but quickly learned that physics was too tangible a field for her. “I really liked all the abstract nonsense in math,” she says. “With physics, you’re too constrained by real-world issues, like gravity, magnetic force. In math, it’s all about creativity and imagination. In math, you’re so much more free.”◾
The American Journal of Mathematics is the oldest continuously published mathematical journal in the Western Hemisphere, established in 1878 at Johns Hopkins University by James Joseph Sylvester, an English-born mathematician and Hopkins professor who also served as the journal’s editor-in-chief from its inception through early 1884. It is published bimonthly by the Johns Hopkins University Press.



